Percentage Change

Percentage change is a fundamental concept in mathematics that quantifies how much a particular value has changed relative to its original amount. Whether it’s an increase or a decrease, understanding percentage change allows us to interpret and compare changes in various contexts, such as finance, business, science, and everyday life. This lesson consolidates the principles of both percentage increase and percentage decrease into a comprehensive guide on percentage change.

Key Definitions

Before delving into calculations, it is essential to understand the key terms involved in percentage change:

• Original Amount: The initial value before any change occurs.
• Final Amount: The value after the change has been applied.
• Amount of Change: The difference between the final amount and the original amount.
• Percent Change: The ratio of the amount of change to the original amount, expressed as a percentage.

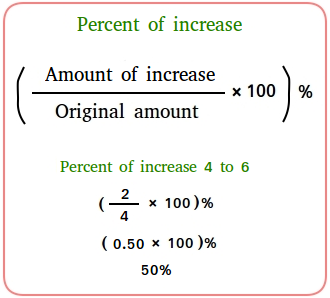
The general formula to calculate percentage change is:

(

Increase/Decrease

|Original Amount|

) × 100

Notice how we take the absolute value of the original value. This formula can represent either a percent increase or a percent decrease:

• Percent Increase: When the final amount is greater than the original amount.
• Percent Decrease: When the final amount is less than the original amount.

Step-by-Step Process

To calculate the percentage change, follow these systematic steps:

1. Identify the Original and Final Amounts: Clearly define the starting (original) and ending (final) values.
2. Determine the Amount of Change:
   • For an increase: Amount of Increase = Final Amount – Original Amount
   • For a decrease: Amount of Decrease = Final Amount – Original Amount

3. Calculate the Ratio of Change to the Original Amount:
   Ratio =
   
   Amount of Change
   
   |Original Amount|
   
4. Convert the Ratio to a Percentage: Percent Change = Ratio × 100
5. Interpret the Result:
   • A positive percentage indicates an increase.
   • A negative percentage indicates a decrease.

Example 1: Calculating Percent Increase

Problem: Calculate the percent increase from 4 to 6.

Solution:

1. Original Amount: 4
2. Final Amount: 6
3. Amount of Increase: 6 – 4 = 2
4. Ratio:
   
   2
   
   4
   
   = 0.5
5. Percent Change: 0.5 × 100 = 50%

Conclusion: The value increased by 50%.

Example 2: Calculating Percent Decrease

Problem: Calculate the percent decrease from 9 to 6.

Solution:

1. Original Amount: 9
2. Final Amount: 6
3. Amount of Decrease: 6 – 9 = -3
4. Ratio:
   
   -3
   
   |9|
   
   = -0.3333
5. Percent Change: -0.3333 × 100 = -33.33%

Conclusion: The value decreased by 33.33%.

Example 3: Negative to Positive Change

Problem: Calculate the percent change from -4 to 8.

Solution:

1. Original Amount: -4
2. Final Amount: 8
3. Amount of Change: 8 – (-4) = 12
4. Ratio:
   
   12
   
   |-4|
   
   = 3
5. Percentage Change: 3 × 100 = 300%

Interpretation: The original negative amount indicates a significant shift from a negative to a positive value, yet a 300% increase relative to the original negative amount.

Example 4: Negative to Negative Change

Problem: Calculate the percentage change from -5 to -10.

Solution:

1. Original Amount: -5
2. Final Amount: -10
3. Amount of Change: -10 – (-5) = -5
4. Ratio:
   
   -5
   
   |-5|
   
   = -1
5. Percentage Change: -1 × 100 = -100%

Interpretation: The value became more negative by 100%, indicating a doubling in the negative direction.

Practical Applications

Understanding percentage change is crucial in various real-world scenarios:

• Finance: Calculating interest rates, investment returns, or changes in asset values.
• Business: Analyzing sales growth, profit margins, or market share changes.
• Science and Engineering: Measuring changes in quantities, concentrations, or other scientific measurements.
• Personal Finance: Tracking salary increases, price changes, or budget adjustments.

Common Misconceptions

Several misconceptions can arise when dealing with percentage changes:

1. Positive Final Value ≠ Always Positive Percentage Change: If the original amount is positive, a positive final value can result in a negative percentage change.
2. Equal Absolute Changes Do Not Guarantee Equal Percent Changes: The same absolute change can represent different percentage changes depending on the original amount.
3. Percent Change Can Be Negative: Negative percentages indicate decreases.

Clarification Examples

• A change from 10 to 15 is a 50% increase, while a change from 20 to 25 is a 25% increase, despite both changes being +5 in absolute terms.
• A change from 9 to 6 is a negative percentage change even though the final value is positive.

Tips for Accurate Calculation

To ensure accurate calculations and interpretations of percentage change:

1. Identify Original and Final Amounts Correctly: Clearly define which value is the starting point and which is the ending point.
2. Handle Negative Values Carefully: Pay attention to the signs and magnitude of the original and final amounts to determine the correct direction of change.
3. Double-Check with Verification: Confirm your calculations by applying the percentage change to the original amount to see if it leads to the final amount.

Verification Example

Problem: Find the percent decrease from 10 to 5.

Solution:

1. Original Amount: 10
2. Final Amount: 5
3. Amount of Decrease: 5 – 10 = -5
4. Ratio:
   
   -5
   
   |10|
   
   = -0.5
5. Percent Change: -0.5 × 100 = -50%

Verification:

Amount of Change = |10| × -50% = 10 × -50% = 10 × -0.5 = -5

Amount of Change = Final Amount – Original Amount

Amount of Change = 5 – 10 = -5

Additional Complex Example

Problem: Calculate the percent change from -10 to 5.

Solution:

1. Original Amount: -10
2. Final Amount: 5
3. Amount of Change: 5 – (-10) = 15
4. Ratio:
   
   15
   
   |-10|
   
   = 1.5
5. Percentage Change: 1.5 × 100 = 150%

Amount of Change = Final Amount – Original Amount

Amount of Change = 5 – -10 = 5 + 10 = 15

Amount of Change = |-10| times 150% = 10 times 1.5 = 15

Conclusion

Calculating percentage change involves understanding the relationship between the original and final amounts, accurately determining the amount of change, and expressing this change as a percentage relative to the original value. While the basic concept is straightforward, complexities arise when dealing with negative values or interpreting the direction of change.

Key Takeaways

• Define Clearly: Always start by clearly defining the original and final amounts.
• Handle Signs Carefully: Pay attention to positive and negative values to accurately interpret the direction of change.
• Simplify Calculations: Use fraction-to-percent simplification techniques for easier computations.
• Verify Your Work: Double-check your calculations to ensure accuracy.

By following the systematic steps outlined above and practicing with various examples, you can master the calculation of percentage changes in diverse scenarios. Whether you’re analyzing financial data, tracking business performance, or managing personal finances, understanding percentage change is an invaluable skill that enhances your ability to make informed decisions based on quantitative changes.